Page 49 - tmp

Chaos, indeed

Никола Милић
Supervisor: Виолета Комненовић
Center for talented youth Belgrade II, Serbia, necromancer0010@gmail.com

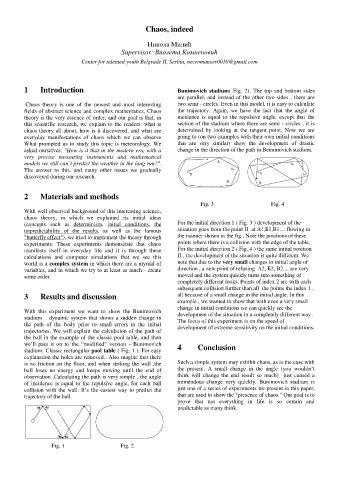
1 Introduction Bunimovich stadium( Fig. 2). The top and bottom sides
are parallel, and instead of the other two sides , there are
Chaos theory is one of the newest and most interesting two semi - circles. Even in this model, it is easy to calculate
fields of abstract science and complex mathematics. Chaos the trajectory. Again, we have the fact that the angle of
theory is the very essence of order, and our goal is that, in incidence is equal to the repulsive angle, except that the
this scientific research, we explain to the readers: what is section of the stadium where there are semi - circles , it is
chaos theory all about, how is it discovered, and what are determined by looking at the tangent point. Now we are
everyday manifestations of chaos which we can observe going to (on two examples with their own initial conditions
What prompted us to study this topic is meteorology. We that are very similar) show the development of drastic
asked ourselves: "How is it that in the modern era, with a change in the direction of the path in Benumovich stadium.
very precise measuring instruments and mathematical
models we still can’t predict the weather in the long run?".
The answer to this, and many other issues we gradually
discovered during our research.

2 Materials and methods
Fig. 3 Fig. 4
With well observed background of this interesting science,
chaos theory, in which we explained its initial ideas
(concepts such as determinism, initial conditions, the For the initial direction 1 ( Fig. 3 ) development of the
unpredictability of the results, as well as the famous situation goes from the point П at А1,Б1,В1.... flowing in
"butterfly effect"), we tried to implement the theory through the manner shown in the fig . Note the positions of these
experiments. These experiments demonstrate that chaos points where there is a collision with the edge of the table.
manifests itself in everyday life and it is through these For the initial direction 2 ( Fig. 4 ) the same initial position
calculations and computer simulations that we see this П , the development of the situation is quite different. We
world is a complex system in which there are a myriad of note that due to the very small changes in initial angle of
variables, and in which we try to at least as much - create direction , a new point of refusing А2, Б2, В2.... are very
some order. moved and the system quickly turns into something of
completely different looks. Points of index 2 are with each
subsequent collision further than all the points the index 1 ,
3 Results and discussion all because of a small change in the initial angle. In this
example , we wanted to show that with even a very small
change in initial conditions we can quickly see the
With this experiment we want to show the Bunimovich development of the situation in a completely different way .
stadium – dynamic system that shows a sudden change in The focus of this experiment is on the speed of
the path of the body prior to small errors in the initial development of extreme sensitivity on the initial conditions.
trajectories. We will explain the calculation of the path of
the ball in the example of the classic pool table, and then
we’ll pass it on to the “modified” version - Bunimovich
stadium. Classic rectangular pool table ( Fig. 1 ). For easy 4 Conclusion
explanation the holes are removed.. Also magine that there
is no friction on the floor, and when striking the wall ,the Such a simple system may exhibit chaos, as is the case with
ball loses no energy and keeps moving until the end of the present. A small change in the angle (you wouldn’t
observation .Calculating the path is very simple , the angle think will change the end result so much) just caused a
of incidence is equal to the repulsive angle, for each ball tremendous change very quickly. Bunimovich stadium is
collision with the wall. It’s the easiest way to predict the just one of a series of experiments we present in this paper,
trajectory of the ball. that are used to show the "presence of chaos." Our goal is to
prove that not everything in life is so certain and
predictable as many think.

Fig. 1 Fig. 2