Page 59 - tmp

GAMES THEORY

MILOŠ ĐURIĆ

ZEMUNSKA 171

INTRODUCTION
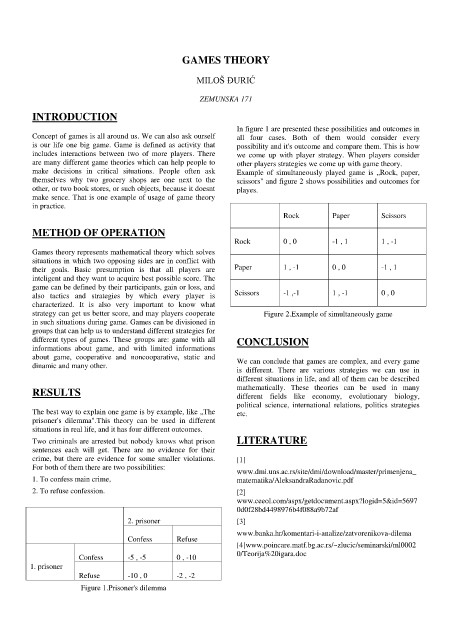
In figure 1 are presented these possibilities and outcomes in
Concept of games is all around us. We can also ask ourself all four cases. Both of them would consider every
is our life one big game. Game is defined as activity that possibility and it's outcome and compare them. This is how
includes interactions between two of more players. There we come up with player strategy. When players consider
are many different game theories which can help people to other players strategies we come up with game theory.
make decisions in critical situations. People often ask Example of simultaneously played game is „Rock, paper,
themselves why two grocery shops are one next to the scissors" and figure 2 shows possibilities and outcomes for
other, or two book stores, or such objects, because it doesnt playes.
make sence. That is one example of usage of game theory
in practice.
Rock Paper Scissors
METHOD OF OPERATION
Rock 0 , 0 -1 , 1 1 , -1
Games theory represents mathematical theory which solves
situations in which two opposing sides are in conflict with
their goals. Basic presumption is that all players are Paper 1 , -1 0 , 0 -1 , 1
inteligent and they want to acquire best possible score. The
game can be defined by their participants, gain or loss, and
also tactics and strategies by which every player is Scissors -1 ,-1 1 , -1 0 , 0
characterized. It is also very important to know what
strategy can get us better score, and may players cooperate Figure 2.Example of simultaneously game
in such situations during game. Games can be divisioned in
groups that can help us to understand different strategies for
different types of games. These groups are: game with all CONCLUSION
informations about game, and with limited informations
about game, cooperative and noncooparative, static and
dinamic and many other. We can conclude that games are complex, and every game
is different. There are various strategies we can use in
different situations in life, and all of them can be described
mathematically. These theories can be used in many
RESULTS different fields like economy, evolutionary biology,
political science, international relations, politics strategies
The best way to explain one game is by example, like „The etc.
prisoner's dilemma".This theory can be used in different
situations in real life, and it has four different outcomes.
Two criminals are arrested but nobody knows what prison LITERATURE
sentences each will get. There are no evidence for their
crime, but there are evidence for some smaller violations. [1]
For both of them there are two possibilities:
www.dmi.uns.ac.rs/site/dmi/download/master/primenjena_
1. To confess main crime, matematika/AleksandraRadanovic.pdf
2. To refuse confession. [2]
www.ceeol.com/aspx/getdocument.aspx?logid=5&id=5697
0d0f28bd4498976b4f088a9b72af
2. prisoner [3]
www.banka.hr/komentari-i-analize/zatvorenikova-dilema
Confess Refuse
[4]www.poincare.matf.bg.ac.rs/~zlucic/seminarski/ml0002
0/Teorija%20igara.doc
Confess -5 , -5 0 , -10
1. prisoner
Refuse -10 , 0 -2 , -2
Figure 1.Prisoner's dilemma